A while ago when you initially considered square attaches and how to tackle radical comparisons, you were presumably acquainted with something many refer to as "the Pythagorean Theorem". This Theorem relates the lengths of the three sides of any right triangle.
The legs of a right triangle (the two sides of the triangle that meet at the right plot) are usually named as having lengths "a" and "b", and the hypotenuse (the long side of the triangle, inverse the right edge) is marked as having length "c". The lengths are connected by the accompanying comparison:
a2 + b2 = c2
This mathematical statement permits you to discover the length of a side of a right triangle when they've provided for you the lengths for the other two sides, and, going in the other heading, permits you to figure out whether a triangle is a right triangle when they've provided for you the lengths for every one of the three sides.






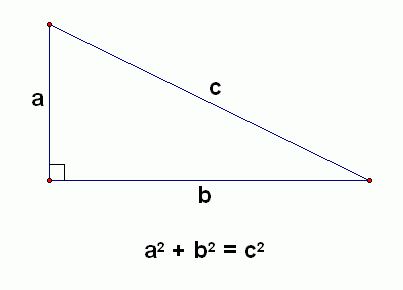
0 comments:
Post a Comment